Quick Look
Summary
On your mark… get set… design! Students discover how sports arenas, rules, and equipment would change if the Olympic Games were conducted on the Moon. During the process, they learn about the engineering design process and physics concepts such as gravity, velocity, acceleration, and friction. They use free online design and programming tools which help them to improve their conceptions and design skills. This activity was developed to be implemented fully online; however, it could easily be adapted for in-class or hybrid classroom use.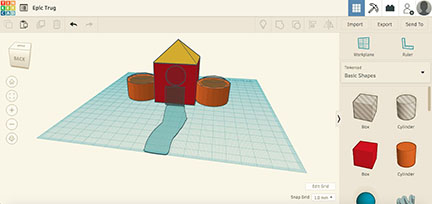
Engineering Connection
The Engineering Design Process is one of the core aspects of engineering. This activity proposes a lunar scenario where the students take the active role as engineers, understanding the effect of physics concepts such as gravity, velocity, acceleration, and friction on the design of arenas, rules, and equipment if the Olympic Games were held on the moon.
Learning Objectives
After this activity, students should be able to:
- Describe how sport arenas, rules, and equipment will change if the Olympic Games are done on the Moon
- Communicate the change of sporting elements (arenas, rules, and equipment) for one chosen sport through sketches
- Recognize that engineers use Computer-Aided Design (CAD) to simulate physics in real-world problems
- Illustrate a real-world situation using TinkerCad
- Examine the concepts of gravity, friction, velocity, and acceleration using computer programming software
- Distinguish basic computer coding structures to simulate the movement of a volleyball
- Identify variables that affect the movement of a volleyball
- Manipulate coding language to understand the effect of forces in a volleyball game on the Moon
Educational Standards
Each TeachEngineering lesson or activity is correlated to one or more K-12 science,
technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN),
a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics;
within type by subtype, then by grade, etc.
Each TeachEngineering lesson or activity is correlated to one or more K-12 science, technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN), a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics; within type by subtype, then by grade, etc.
NGSS: Next Generation Science Standards - Science
| NGSS Performance Expectation | ||
|---|---|---|
|
MS-ETS1-1. Define the criteria and constraints of a design problem with sufficient precision to ensure a successful solution, taking into account relevant scientific principles and potential impacts on people and the natural environment that may limit possible solutions. (Grades 6 - 8) Do you agree with this alignment? |
||
| Click to view other curriculum aligned to this Performance Expectation | ||
| This activity focuses on the following Three Dimensional Learning aspects of NGSS: | ||
| Science & Engineering Practices | Disciplinary Core Ideas | Crosscutting Concepts |
| Define a design problem that can be solved through the development of an object, tool, process or system and includes multiple criteria and constraints, including scientific knowledge that may limit possible solutions. Alignment agreement: Analyze and interpret data to provide evidence for phenomena.Alignment agreement: Use mathematical representations to describe and/or support scientific conclusions and design solutions.Alignment agreement: | The more precisely a design task's criteria and constraints can be defined, the more likely it is that the designed solution will be successful. Specification of constraints includes consideration of scientific principles and other relevant knowledge that is likely to limit possible solutions. Alignment agreement: | The uses of technologies and any limitations on their use are driven by individual or societal needs, desires, and values; by the findings of scientific research; and by differences in such factors as climate, natural resources, and economic conditions. Alignment agreement: |
| NGSS Performance Expectation | ||
|---|---|---|
|
MS-PS2-4. Construct and present arguments using evidence to support the claim that gravitational interactions are attractive and depend on the masses of interacting objects. (Grades 6 - 8) Do you agree with this alignment? |
||
| Click to view other curriculum aligned to this Performance Expectation | ||
| This activity focuses on the following Three Dimensional Learning aspects of NGSS: | ||
| Science & Engineering Practices | Disciplinary Core Ideas | Crosscutting Concepts |
| Science knowledge is based upon logical and conceptual connections between evidence and explanations. Alignment agreement: Apply scientific ideas or principles to design an object, tool, process or system.Alignment agreement: Laws are regularities or mathematical descriptions of natural phenomena.Alignment agreement: | Gravitational forces are always attractive. There is a gravitational force between any two masses, but it is very small except when one or both of the objects have large mass—e.g., Earth and the sun. Alignment agreement: | Models can be used to represent systems and their interactions—such as inputs, processes and outputs—and energy and matter flows within systems. Alignment agreement: |
Common Core State Standards - Math
-
Use appropriate tools strategically.
(Grades
K -
12)
More Details
Do you agree with this alignment?
-
Model with mathematics.
(Grades
K -
12)
More Details
Do you agree with this alignment?
International Technology and Engineering Educators Association - Technology
-
Students will develop an understanding of the attributes of design.
(Grades
K -
12)
More Details
Do you agree with this alignment?
-
Students will develop an understanding of the relationships among technologies and the connections between technology and other fields of study.
(Grades
K -
12)
More Details
Do you agree with this alignment?
State Standards
Indiana - Math
-
Use appropriate tools strategically.
(Grade
7)
More Details
Do you agree with this alignment?
-
Model with mathematics.
(Grade
7)
More Details
Do you agree with this alignment?
Indiana - Science
-
Identify a need or problem to be solved.
(Grades
K -
8)
More Details
Do you agree with this alignment?
-
Present evidence using mathematical representations like graphs and data tables.
(Grades
6 -
8)
More Details
Do you agree with this alignment?
Materials List
If the activity is online, each student will need:
Each student needs:
- Laptop, mobile phone, tablet or desktop with access to internet for video calls (with group chat capability), YouTube, TinkerCAD, and Octave (https://octave-online.net/)
- a TinkerCAD account (free)
- Code (Day 3 – Step 4) attachment (for Day 3)
- paper
- pencils, pens, and/or markers
Students also need access to a central class social media platform or learning management system for the post-activity assessments.
Optional: Teachers may use a document camera to show written demonstrations to the class. This activity can be done in groups or individual (with enough tablets for each student) in person.
Worksheets and Attachments
Visit [www.teachengineering.org/activities/view/pur-2576-lunar-olympics-physics-online-activity] to print or download.Pre-Req Knowledge
1. A basic understanding of Newtonian mechanics, parabolic motion, gravity, friction, acceleration, and velocity; 2. Basic computational skills, 3. Basic drawing skills
Introduction/Motivation
For many centuries humans have seen the sky and dreamt of going to the Moon. It took until 1969 for the first human to put their foot on its surface; however, since 1972, no other person has gone back. Recent efforts consider the Moon as a place to restart a dynamic space mission, using it as place to explore, learn, and even live. Can you imagine that!? What would we have to do to live on the moon? We would have to rethink many things in our daily lives. For example, growing food, transportation to school, or playing a sport to stay healthy. With time, we would also replicate some part of our culture, like going to the movies, to an amusement park, or to sporting events. Can you imagine how the Olympic Games would be done on the Moon?
The following activity is focused on how we might think about conducting the Olympics on the Moon. How might we need to change the equipment, arenas, or rules? To answer this question, we will use our understanding of some physics concepts like gravity, velocity, acceleration, and friction along with our knowledge of sporting events to think how we, as engineers, could redesign aspects of the competition. In this activity, we will learn about various Olympic sports and think about how changes in environment (from Earth to Moon) would impact the sports and then design new solutions to help the athletes compete safely and fairly on the Moon. You will simulate physical phenomenon by doing some computer programming to visualize how a ball may travel differently on the moon. This challenge asks you to engineer the first Olympics on the Moon, are you ready?
Procedure
Background
The following activities highlight how engineers require different kinds of knowledge for designing a way for humans to live on the Moon. As lunar habitants, we would bring some Earth cultural activities to the Moon, and the Olympics could be one of them. What would be different about cultural activities on the Moon from those done on the Earth? Essentially, the Moon is Earth’s satellite, meaning that it is smaller. This difference in the size impacts some natural forces, gravity being the most evident. For these activities, gravity is conceived as an attraction force that an object exerts on other. It means that a big object like Earth has more attraction force than a smaller one, like the Moon. In other words, Earth has a higher attraction force than the Moon. These activities emphasize this aspect and its impact on developing the Olympics on the Moon. On Earth, gravity is 32.2 ft/s2 while on the Moon it is of 5.37 ft/s2 (one sixth of Earth’s gravity). It means that physical phenomena such as velocity, acceleration, or friction will change, which affects the rules, gear, and arenas of all the sports. For example, sports related to swimming pools or equestrian sports would not be done. The weaker gravity wouldn’t allow the water or horses to be on the ground; they would float.
The proposed activities tackle the concepts of gravity, friction, velocity, and acceleration, using the Newtonian mechanics logic for parabolic motion in Volleyball as an example. The following link provides more information about the equations associated to this phenomenon and how to use them (https://chitowntutoring.com/projectile-motion-kinematics-in-2-dimensions/). Finally, the concepts of Computer Aided Design (CAD) and coding are introduced using two free online software tools (TinkerCad and Octave) that will help the students develop design and computational skills.
Part 1 (Day 1)
Before the Activity
- Set up a class chat or social media where students can share their thoughts and ideas.
- Have the students:
- Watch “Overview of the Olympics for Kids” - https://www.youtube.com/watch?v=xBsRx4wN_v4
- Read about the Olympics: https://forkidsplus.com/list-of-sports/
- Write down their 3 favorite individual sports and 3 favorite team sports (students may draw from their own knowledge or choose sports from the reading)
- Think about: How would the sports change if the Olympics are done on the moon?
Step 1. Introduction Day 1 (5 minutes)
Summarize the content of the pre-session video, discussing the following questions with the kids (Example answers are given):
- What is the history of the Olympics? (Example answers include: began in Greece in 776 BC, started with only one sport (200 m sprint) and then more sports got added over time, happens every 4 years, stopped for a while and then restarted in 1896, etc.)
- Why do the Olympic Games exist? (Example answer: To foster world peace and friendly competition.)
- How have the Olympics’ sports changed with new technology? (Example answer: More sports are included in the Olympics that would not be possible without new technology.)
Step 2. Problem Statement (5 minutes)
After the discussion, propose the following problem:
Imagine that the International Olympic Organization and NASA hired you, as engineers, to design the arenas, game rules, and gear for doing the first Olympics on the Moon. For that, you have to answer the following questions:
- How will sports change if the Olympics are done on the moon?
- Would the venues be different?
- Would the game rules be different?
- Would the athletic equipment be different?
You can write these questions in the chat and ask them to write them on a piece of paper.
Step 3. Group Activity (15 minutes)
Together, make an arena, rules, and equipment for American football (as an example because American Football is not an Olympic sport). If you prefer, you can use another sport such baseball or rugby since they are Olympic sports (or another sport that is familiar to the students). Focus your camera on a paper and drawing and begin to sketch a design with help from the students. Take their suggestions and incorporate into a rough, new design. It is not necessary to have great drawing skills, the goal is to be able visualize and communicate the ideas. Good labeling can make up for some drawing mistakes. You can encourage the students’ participation using the following questions:
- What is it like on Earth?
- How would it be different on the Moon?
- How far would the ball go on the moon?
- How would the trajectory change?
Figures 1 and 2 show the result of one guided drawing.


Step 4: Individual Activity (20 minutes)
Ask the students to choose a new sport and write its name on a piece of paper as a title. On the same piece of paper, ask them to:
- Write down the rules of that sport on the Moon, and
- Sketch a new venue and the athletic equipment or gear for that sport on the Moon
Step 5: Share! (5 minutes)
Have each student share and explain their new arena and rules for their sport. If you like, show an example, previously made by you, if the students are shy and hesitant to share theirs.
Step 6: Post-Session Activity
If the kids do not finish their drawings, ask them to finish and upload it in a group social media for the class with help of their parents or guardians. Also, ask them to create a free TinkerCAD account (https://www.tinkercad.com/) to prepare for the next session.
Part 2 (Day 2)
Before the Activity
- Ensure that you know how to use TinkerCAD to design a simple house (to model it for the students in Step 3). If you don’t know how to use this site, please refer to the following video “Getting Started in Tinkercad: A Tutorial for Complete Beginners” to learn and practice some skills before teaching the session: https://www.youtube.com/watch?v=60xfIu-lqAs.
Step 1: Share! (3 minutes)
Have the students share what they have worked on since the previous session. You can also give a recap of the previous session and show the pictures that were posted on the activity’s social media.
Step 2: Introduction Day 2 (3 minutes)
Discuss with students the following questions (Example answers are given):
- What is Computer-Aided Drawing (CAD)? (Example answers: CAD uses computers to create, modify, and analyze a 2D or 3D design.)
- Why is CAD useful? (Example answers: Engineers can save time and resources by testing and optimizing a design virtually rather than fabricating and testing multiple prototypes.)
- How is CAD used for modeling before building? (Example answers: Engineers can use CAD to develop blueprints or provide uniform measurements to guide the building of a design.)
In case they do not know the answers, you can explain to them in accordance to the definition made in the glossary.
Step 3: Demonstration (10 minutes)
- Ask the students to draw a 3D drawing of a house on paper (5 minutes).
- Have students share their drawings in front of the camera (2 minutes).
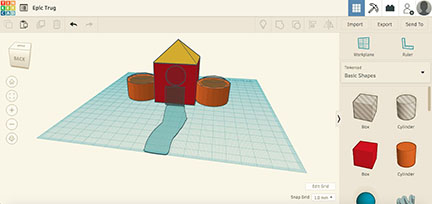
- Show the students TinkerCAD and walk them through how to design one house together. For that, model the elements of the house using a combination of the students’ drawings (roof shape, backyard, front door). Let the students choose the shapes and colors for the instructor to use (10-15 minutes). Figure 3 shows a possible design of a house as part of the demonstration.
Step 4: Physics Review (10 minutes)
Explain the relationships of the relevant properties between the Earth and the Moon:
- Gravity
- There is less gravity on the moon, approximately one-sixth that on Earth
- Common misconception: “There is no gravity on the moon” (false)
- Correction: “There is less gravity on the moon than there is on Earth” (true)
- Friction/air resistance
- The moon has no atmosphere. Therefore, there will be no air resistance to slow down moving objects.
- Velocity
- Projectiles on the moon will travel further and faster because there is no air resistance and less gravity.
- Common misconception: “Heavier objects fall faster than lighter objects” (false)
- Correction: “Objects with less surface area fall faster than objects with more surface area” (true)
- Acceleration
- Acceleration due to gravity is much less on the Moon than it is on Earth. Acceleration is change of velocity, which will happen at a slower rate as objects fall down.
If students are still confused, show the following video “Danger! Falling Objects” - https://www.youtube.com/watch?v=dxcx35x5L9Y. Also, you can do a demo dropping two objects with the same mass from chest-high (Pencil and plastic bag, flat sheet of paper and crumpled up paper ball).
Step 5: Drawing Exercise (10 minutes)
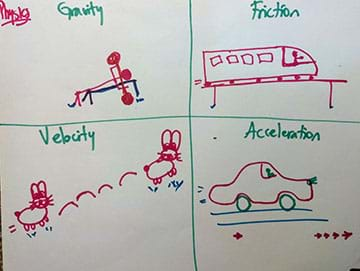
Ask the kids to take a piece of paper and separate it into four quadrants. In each corner, label one of the following terms: Gravity, Friction, Velocity, Acceleration. Have each student draw a picture in each box what that term represents to them. Finally, ask to share their drawings using the video camera. Figure 4 shows the result of this activity.
Step 6: Hypothesize (10 minutes)
Have the kids sketch a volleyball arena on another piece of paper (Figure 5). (If students are unfamiliar with volleyball, inform them that it is a team sport where the goal is to hit the ball over the net and the other team tries to prevent it from hitting the ground.) This arena represents a cartesian plane with the y (vertical) axis showing the height, and the x (horizontal) axis showing the distance. Then ask them to sketch the trajectory of two or three of the following scenarios that you have picked previously:
What would the path of a volleyball, served over a net look like...
- If it was served underhand?
- If it was served overhand?
- If it was served in a vacuum (no air)?
- If it was served on the moon?
- (Optional question #1 Ask if students are familiar with volleyball) if it was served by someone 1.5 meters feet tall? By someone 2 meters tall?
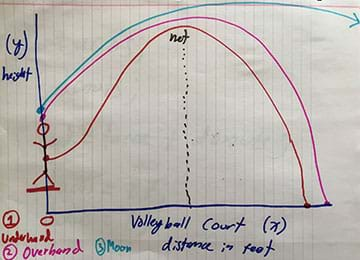
Note: The graph is a general sketch to illustrate the concepts. However, it uses the cartesian coordinates (X and Y) as means to introduce them later (Day 3) to the equations during the code modification.
Step 7: Share! (4 minutes)
Have each student share what they think the trajectory would look like for every scenario and notice the differences between the types of serves and the various conditions. Use the following question to guide the discussion: How does this graph relate to the physics terms we discussed earlier?
Step 8: Post-Session Activity
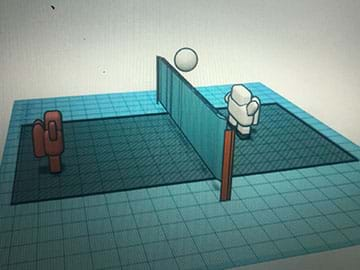
If the kids could not finish their drawings of gravity, friction, velocity, and acceleration, they have the opportunity to do so now and post them online. Also, ask the kids to use TinkerCad to create a volleyball court and upload the screenshot of the result. Encourage students to research the dimensions of a volleyball court 9 m x 18 m (29 ft, 6 in x 59 ft) and net height 2.4 m (8 ft) for men and 2.2 m (7 ft 4 in) for women) and to draw the court (and any players) to scale. Figure 6 shows a TinkerCad design done by some students as a reference.
The idea with this step is that the students could improve their ability using TinkerCAD by themselves, at home. Initially, the students could pay attention to both of the details, arena’s size and gears, it is according to their desire. However, if the teacher considers, it is possible to focus the attention on one of them, in case that it will be used as part of the assessment process. In our opinion, if the option is broad, the students can create more conceptual sketches that can combine the obtained learnings. It is also possible to expand the step, asking the students to sketch the volleyball court and gears according to what they learned during the day solve the confusion.
Part 3 (Day 3)
Before the Activity
- Check that students can access Octave (https://octave-online.net/) on their laptops or computers.
- Download the Code (Day 3 – Step 4) attachment.
- Ask the kids to watch this video “What is Coding?” - https://www.youtube.com/watch?v=N7ZmPYaXoic. Also, ask them to answer the following questions on paper for discussing them during the session:
- What is coding? (Possible answer: the process of designing and building an executable computer program to accomplish a specific computing result or to perform a specific task.)
- How does a computer know what to do? (Possible answer: it reads the very detailed code and follows the directions; the code gets translated into binary 1’s and 0’s which results in various outputs like MS Word and iTunes)
- How is writing computer code similar to:
- A chef writing a recipe?
- You giving your friend directions to your house?
- You teaching a dog to “sit” or “shake”?
(Possible answer: all of these situations involve writing or giving a specific set of instructions and having someone follow the instructions, they all are creating something out of simple inputs)
Step 1: Share! (5 minutes)
Before starting the activity, ask the students to share their work since the last session: What have you worked on since yesterday’s session? If you see they do not want to talk, show your TinkerCAD volleyball court (Figure 6) and discuss it instead.
Step 2: Introduction (5 minutes)
Discuss the questions proposed in Day 3 Before the Activity with the students. Ask as many students as you can to answer the questions. In case they do not know the answers, you can explain them and refer to the vocabulary definitions.
Step 3: Problem Statement (3 minutes)
After the discussion, propose the following question: How can we use a computer to help us visualize the Olympic games rules for various sports?
You can write this question in the chat or ask them to write it on a piece of paper.
Step 4: Introduction to Coding (10 minutes)
- Before starting the demonstration, ask the kids about the definition of a variable. If they do not know about it, you can use the one found in the glossary. If they know, you can make more questions about where they find variables or what kind of variables they know. Also, you can summarize the definition of variable as “a symbol that represents a value” for closing this discussion.
- Now, you can explain how you can assign values to variables inside a code following this process:
- Focus the camera on a piece of paper (or write on a blackboard if doing the activity in-person), write the following equation: food = pizzas + cakes (you can use another food, or ask the students to tell you one or more)
- Assign a number to each word of food: pizzas = 4; cakes = 3 (ask the kids for numbers if you want)
- Complete the simple math equation in front of them and show the result (food = 4 + 3 = 7)
- Focus the students’ attention to the screen of your laptop (both online and in-person) and open Octave (https://octave-online.net/), and write the following lines:
pizzas = 4; (enter)
cakes = 3; (enter)
food = pizzas + cakes; (enter)
- Edit any of the values from the previous statements and the values will update. You can ask the students to suggest numbers to watch the change.
- Stay in Octave to explain how coding can be used for formulas. For that, demonstrate its use with a simple formula that students are familiar with, such as area of a rectangle (area = weight * height) or a circle (area = pi * radi^2)
Step 5: Coding Demonstration (10 minutes)
- In Octave, use the code found in the Code (Day 3 – Step 4) attachment, for simulating a volleyball serve with the given conditions.
- Show how you can change the value of the variables at the beginning (and not worry about the rest of the code) to have the computer do something completely different without re-writing the code every time. Let the students pick numbers and variable names.
Step 6: Individual/Group activity (13 minutes)
- Have students go to Octave (https://octave-online.net/), This activity could be done individually (online) or by groups (in-person), according to the amount of equipment you have.
- Have the students write what the next line would be in the previous code (building on the Code attachment),or write the code for a similar equation. If the kids do not know where to start, you can suggest using the equations for free fall (h = 1/2gt2, and v = gt).
Step 7: Share! (4 minutes)
Have each student share observations of the process. You can use the questions:
- Could you write the code for an equation? Did it work?
- How did you feel creating the code?
Step 8: Post-Session Activity
Ask the kids to modify the code using https://octave-online.net/ for serving a tennis ball on a tennis court on Earth and on the Moon. (If students are unfamiliar with tennis, explain this this sport is similar to volleyball in that the goal is to get a ball over a net to hit the ground on the other side; however, there are less players and they hit the ball with a racket). They can answer the following questions in a piece of paper, notebook, or social media used for the activity:
- What variables need to change (court length, player height, gravity, net height, ...)?
- Can you make the ball go over the net and land at exactly 23.5 m (~77 feet)?
- What serving speed and angle is required to barely clear the net and land as close to the back line as possible?
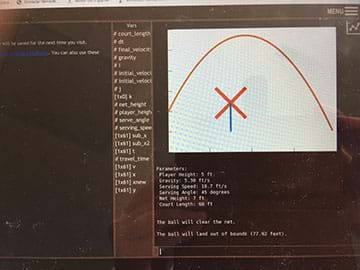
Tell them that if they need more help to understand how to use the site, watch the following instructional video “PALS Engineering Coding demo” - https://youtu.be/UdG_oD-GxMs. Ask them to post their findings and online output (screenshot of the result – Figure 7). Finally, ask them that submit the answers for the following questions:
- What kind of connection did you notice between the activities and the work of engineering?
- What did you learn from the activities?
- After these activities, what do you think you need to learn more about doing the Olympics on the Moon?
Vocabulary/Definitions
acceleration: Rate of change of the velocity of an object with respect to time.
computer programming (coding): Process of designing and building an executable computer program to accomplish a specific computing result or to perform a specific task.
computer-aided design (CAD): Form of 3D modeling, often in the form of software such as TinkerCAD, Autodesk Inventor, and Autodesk Fusion360.
friction: Force resisting the relative motion of solid surfaces, fluid layers, and material elements sliding against each other.
gravity: Natural phenomenon by which all things with mass or energy—including planets, stars, galaxies, and even light—are brought toward (or gravitate toward) one another. On Earth, gravity gives weight to physical objects, and the Moon's gravity causes the ocean tides.
moon: An astronomical body orbiting Earth and is the planet's only natural satellite.
Olympic Games: Athletic festival that originated in ancient Greece and was revived in the late 19th century.
variable: Symbol which functions as a placeholder for varying expression or quantities, and is often used to represent an arbitrary element of a set. In addition to numbers, variables are commonly used to represent vectors, matrices and functions.
velocity: Rate of change of its position with respect to a frame of reference, and is a function of time.
Assessment
Pre-Activity Assessment
Introduction: The Introduction steps (Step 1 – Days 1 and 2, and Step 2 – Day 3) are the pre-activity assessment. The idea of these instructions is, through the use of questions, to identify the prior knowledge of the students about the content that will be taught. If the students do not know the answer, the teacher may share the definitions shown in the glossary or elicit the definitions in another way.
Activity Embedded (Formative) Assessment
Share: Use the Share steps (Step 5 – Day 1, and Step 7 – Days 2 and 3) to ask the students to share their individual or group work. Through the questions posted in these steps, foster a discussion between the students, asking questions such as: Does anyone want to give a respectful opinion about what __ (name)__ showed? Did you do the same? What was different? Why? The idea is to see if all the students are handling the same concepts as well as to scaffold those students who did not understand the concepts, while listening to others and the teacher or sharing their results.
Post-Activity (Summative) Assessment
Post-Session Activities: Each day has a post-session activity which encourages students to learn outside the classroom or virtual session. They consist of a to-do activity where the student applies the knowledge constructed during the session and communicates their results on the platform the teacher uses for communicating with students (this could be a social media platform or on a site like Google Classroom). For Days 1 and 2, the post-session activity is focused on motivation. In that sense, the assessment is focused on whether the results were uploaded/sent or not. For Day 3, besides uploading/sending the screenshot of the results after modifying the coding in relation to some questions (posted the procedure section Day 3 – Post-session activity), the students are encouraged to reflect about their learning. For that, teachers can also use the following questions:
- What kind of connection did you notice between the activities and the work of engineering?
- What did you learn from the activities?
- After these activities, what do you think you need to learn more about doing the Olympics on the Moon?
Investigating Questions
Although each day has specific questions, the general essential questions are:
- How will sports change if the Olympics are done on the moon?
(Answer: Gravity is the most important factor to consider in case that the Olympics will be done on the Moon. Many of the sports will have to change their rules, gear, and arenas to maintain similarities with the sports on the Earth. Some of the sports couldn’t be done on the Moon. For example, swimming would not be possible because it would require sufficient gravity to maintain water in a pool.)
- Would the arenas/courts be different?
(Answer: Yes. Each sport has specifications related to the size of its arenas. It is related to some physical variables (velocity, acceleration, and friction) that are proportional to gravity. As Moon’s gravity is one sixth of that of Earth’s, those parameters would change, impacting on the design and construction of the arenas.)
- Would the game rules be different?
(Answer: Yes. Gravity would impact on how each movement will be executed by the athletes. Balls and arrows could be thrown further, movements would be slower, jumps would be higher, etc. In that sense, rules would change to create a fair competition environment.)
- Would the gear/clothing be different?
(Answer: Yes. Gravity would impact on athletes’ performance. Water and objects would float and some skills would be affected, such as jumping, throwing, or running. Therefore, special gear and equipment would be required to allow breathing since the Moon has no atmosphere.)
Troubleshooting Tips
It is possible that during the Share your Work moments, the kids do not want to talk, especially online. In that case, it is useful to show them what was your solution to the problem. It helps them to feel more confident to talk and show the results of their designs and learnings. If the activity is done online, we suggest having two instructors for continuing the session in case of one of them cannot continue because an internet or energy outage.
Activity Extensions
Post-session activities in each day are both activity extensions and assessment activities. Please look in the procedure and assessment sections for more details.
Activity Scaling
Have more advanced students experiment with TinkerCAD and Octave. If the school has a 3D printer it is possible to print the design of students who made the best designs in TinkerCAD.
Additional Multimedia Support
- History of the Olympics: https://www.youtube.com/watch?v=xBsRx4wN_v4
- List of the Olympics sports: https://forkidsplus.com/list-of-sports/
- Velocity, acceleration, and friction: https://www.youtube.com/watch?v=dxcx35x5L9Y
- Computer programming: https://www.youtube.com/watch?v=N7ZmPYaXoic
Subscribe
Get the inside scoop on all things TeachEngineering such as new site features, curriculum updates, video releases, and more by signing up for our newsletter!More Curriculum Like This

Imagining themselves arriving at the Olympics gold medal soccer game in Rio, Brazil, students begin to think about how engineering is involved in sports. After a discussion of kinetic and potential energy, an associated hands-on activity gives students an opportunity to explore energy-absorbing mate...

Students are introduced to the concepts of force, inertia and Newton's first law of motion: objects at rest stay at rest and objects in motion stay in motion unless acted upon by an unbalanced force. Students learn the difference between speed, velocity and acceleration, and come to see that the cha...

High school students learn how engineers mathematically design roller coaster paths using the approach that a curved path can be approximated by a sequence of many short inclines. They apply basic calculus and the work-energy theorem for non-conservative forces to quantify the friction along a curve...
References
Chitowntutoring.com. (Accessed September 12, 2020.) (Source of parabolic motion equations and theory) https://chitowntutoring.com/projectile-motion-kinematics-in-2-dimensions/ (Accessed September 12, 2020.)
Ross, A. (2013). If the Olympics were held on the Moon. September 26, 2013. https://prezi.com/eeszd93atptd/if-the-olympics-were-held-on-the-moon/
Copyright
© 2021 by Regents of the University of Colorado; original © 2020 Purdue UniversityContributors
Andrew Gray, Undergraduate student; Cristián Vargas-Ordóñez, Graduate student; Dr. Morgan Hynes, SupervisorSupporting Program
Purdue-CISLUNAR Initiative, Purdue UniversityAcknowledgements
This curriculum was based upon work supported by the National Science Foundation under RET grant no. EEC 1454152—CAREER: Broadening Contexts to Motivate Participation in Engineering through the Purdue University-CISLUNAR Initiative. Any opinions, findings, and conclusions or recommendations expressed in this material are those of the authors and do not necessarily reflect the views of the National Science Foundation.
Last modified: September 17, 2021









User Comments & Tips