Quick Look
Grade Level: 9 (9-10)
Time Required: 45 minutes
Expendable Cost/Group: US $7.00 This activity also uses some non-expendable (reusable) items such as calculators and laser pointers; see the Materials List for details.
Group Size: 4
Activity Dependency:
Subject Areas: Geometry
NGSS Performance Expectations:

| HS-ETS1-2 |

Summary
Students explore in detail how the Romans built aqueducts using arches—and the geometry involved in doing so. Building on what they learned in the associated lesson about how innovative Roman arches enabled the creation of magnificent structures such as aqueducts, students use trigonometry to complete worksheet problem calculations to determine semicircular arch construction details using trapezoidal-shaped and cube-shaped blocks. Then student groups use hot glue and half-inch wooden cube blocks to build model aqueducts, doing all the calculations to design and build the arches necessary to support a water-carrying channel over a three-foot span. They calculate the slope of the small-sized aqueduct based on what was typical for Roman aqueducts at the time, aiming to construct the ideal slope over a specified distance in order to achieve a water flow that is not spilling over or stagnant. They test their model aqueducts with water and then reflect on their performance.Engineering Connection
Aqueducts are an ancient form of transporting water, and their structural systems are still in place today, although no longer in use. Two thousand years ago when Romans built aqueducts, the objective was to transport fresh water over miles and miles of land to reach the cities. They built tall and sturdy structures with built-in channels for water to flow from its source all the way to the aqueduct end. The structures were carefully designed so the flow rate would not drain the source too quickly, while also not letting the water stand still. To ensure optimum flow rate, the channels were engineered to only drop several inches every 100 feet. Today, we are accustomed to transporting water through underground pipes using gravity and pumps, but 2000 years ago, the technology of aqueducts and capabilities were absolutely amazing and nothing like them had ever been seen or built before.
Learning Objectives
After this activity, students should be able to:
- Identify geometrical shapes and patterns associated with engineering design.
- Use the dimensions of geometrical shapes such as triangles to find the dimensions of different shapes.
Educational Standards
Each TeachEngineering lesson or activity is correlated to one or more K-12 science,
technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN),
a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics;
within type by subtype, then by grade, etc.
Each TeachEngineering lesson or activity is correlated to one or more K-12 science, technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN), a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics; within type by subtype, then by grade, etc.
NGSS: Next Generation Science Standards - Science
| NGSS Performance Expectation | ||
|---|---|---|
|
HS-ETS1-2. Design a solution to a complex real-world problem by breaking it down into smaller, more manageable problems that can be solved through engineering. (Grades 9 - 12) Do you agree with this alignment? |
||
| Click to view other curriculum aligned to this Performance Expectation | ||
| This activity focuses on the following Three Dimensional Learning aspects of NGSS: | ||
| Science & Engineering Practices | Disciplinary Core Ideas | Crosscutting Concepts |
| Design a solution to a complex real-world problem, based on scientific knowledge, student-generated sources of evidence, prioritized criteria, and tradeoff considerations. Alignment agreement: | Criteria may need to be broken down into simpler ones that can be approached systematically, and decisions about the priority of certain criteria over others (trade-offs) may be needed. Alignment agreement: | |
Common Core State Standards - Math
-
Apply geometric methods to solve design problems (e.g., designing an object or structure to satisfy physical constraints or minimize cost; working with typographic grid systems based on ratios).
(Grades
9 -
12)
More Details
Do you agree with this alignment?
International Technology and Engineering Educators Association - Technology
-
Students will develop an understanding of engineering design.
(Grades
K -
12)
More Details
Do you agree with this alignment?
Materials List
Each group needs:
- 40 Popsicle sticks
- 30 half-inch cube blocks; such as a bag of 100 half-inch blocks for $5 from Woodworks Ltd.
- long water channel, about 3-feet long; for example, use long, plastic hot wheels tracks or premade wooden channels and hot glue to connect the channels; length is up to the teacher, but 3 feet is a good starting point
- laser pointer
- yardstick
- 12-inch ruler
- calculators
- paper and pencils
- hot glue gun and glue sticks
- 1 wooden baseboard that is 6-12 inches wide x the water channel length
- measuring cup and water, to carry water that is poured into the aqueduct
- cup or bucket, to catch water at the end of the aqueduct
- Arch Calculations Worksheet, one per group
- How to Build an Arch Handout, one per group
- (optional) plastic bag or box, in which to contain each group’s materials, for easy distribution
Worksheets and Attachments
Visit [www.teachengineering.org/activities/view/cub_aqueducts_lesson01_activity1] to print or download.Pre-Req Knowledge
- Students must know about trigonometric ratios (law of sines, cosines and tangents.)
- Students must know the Pythagorean Theorem and how it can be used.
Introduction/Motivation
Think of the last time you walked on a bridge or saw a bridge of some sort. Do you recall what was supporting that bridge and keeping it from collapsing under your feet? Typically, engineers these days design bridges with support beams both under and above bridges for provide maximum safety. However, more than 2000 years ago when the Romans controlled a vast empire, they used an approach that was extremely innovative for its time—something that we today call the Roman arch.
The Romans created a design that enabled a structure to be much stronger (and thus safer) than anything previously seen before while using less material. These arches were so innovative and useful (and aesthetically pleasing) that almost every piece of Roman architecture involved an arch of some sort. These arch designs are still used today and can be seen in architecture around the world.
In this activity, your engineering challenge is to build a small-sized model aqueduct to transfer water from one point to another. You will design arches similar to the way the Romans did in order to support the channel carrying the water. Design your aqueduct in a way that lets water flow through the channel to the other end easily with little to no water spilling.
Why did ancient—and modern—communities build water transport systems? (See if students have ideas.) Sometimes local water sources are polluted or taste bad because of minerals in the water. Aqueducts—and modern water treatment distribution systems—are used to move clean and fresh water from a distant location to where it is needed. You may have heard of water quality and health problems in Flint, Michigan. In that town, the municipal water supply caused pipes to release lead into the tap water, so eventually the city changed its water source and treatment method. The Romans primarily used concrete, along with stone and bricks, for their water transport systems, which avoided the negative health effects of lead.
Procedure
Before the Activity
- Make copies of the Arch Calculations Worksheet and How to Build an Arch Handout, one each per group. The worksheet can be completed individually, but to save time it is recommended to let students work on it as a group.
- Gather materials. It helps to organize each group’s materials into individual plastic bags or boxes, and place those materials at a table in the classroom.
With the Students
- Administer the pre-activity assessment, as described in the Assessment section.
- Present to the class the Introduction/Motivation content to introduce the engineering challenge.
- Briefly explain to the class that they will be completing a worksheet to help them design and build Roman arches. Then hand out the worksheet and have students complete the calculations to answer both problems.
- Divide the class into groups of four students each. (Groups can be larger or smaller, depending on the amount of materials.)
- Ask one person from each group to get the materials for his/her group.
- Show students how they can use each of the tools. The laser pointer can extend a level surface to a wall so after students have calculated the drop in height of their aqueducts, they can scale and measure them using the laser as a reference point. The Popsicle sticks are useful for building the support structures and to slightly increase the arch height to achieve the perfect support amount for the aqueduct channel.
- Have students first figure out and then build arch support structures as a necessary part of building their model aqueducts to the planned dimensions. The support structure is a temporary wooden framework that supports the arch while it is being constructed and then is removed once the arch is closed and self-supporting. Direct the groups to each make one arch support structure using only Popsicle sticks, which they will re-use in the construction of all of their arches. Also alert students to be careful with the support structure and not break it since it is very important.
- Give students the constraints for building their aqueducts from point A to point B. The constraints are based on the length of the aqueduct channel. Also give students a required number of arches they need to build to hold up the bridge. For example, for a three-foot aqueduct, six arches are needed, or two arches per foot.
- Have students begin calculations on the slope needed for the aqueduct. Use the example of an aqueduct dropping 0.5 inches every three feet.
- Once students have finished their calculations, direct them to begin building the aqueducts. To build each arch, students need the number of blocks according to their worksheet calculations, plus blocks to place under the arches to adjust the aqueduct height. Give groups the how-to-build handout to help them build the arches.
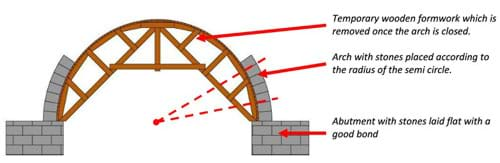
- Direct students to glue their arches to the baseboard at distances they have calculated. For example, if building a three-foot channel with three six-inch-diameter arches, then the arches require gaps between them in order to spread them out evenly. The baseboard serves as the “land” on which the arches are positioned/attached. The placement location on the baseboard will vary by team because it depends on the arch size.
- Once students are done building, one group at a time, place a group’s setup of arches on a counter or floor, positioned with a catch bucket at the end. Then pour water into the beginning (high end) of the aqueduct and observe its flow to the other end.
- Collect the students’ work—model aqueducts and calculations—and ask them to write their names on them.
- Lead a class discussion about how each group went about building its arches and the resulting performance of its aqueduct. Ask the students: What worked? What did not work? (Points to make: Ideally, aqueducts are strong and maintain their structural integrity while in use. Ideally, the water flow is not so fast that it splashes out of the channel, but not so slow as to make the water stagnant.) How would you improve your aqueduct? What process did you go through to make sure your measurements were correct? What could you have done differently to speed up the process?
Vocabulary/Definitions
aqueduct: A bridge structure built to transport water from a point A to a point B.
arcade: A series of adjoining rounded arches.
arch: A curved architectural structure that spans a space and supports the weight it carries.
keystone: The central and final stone placed in the arch, located in the top center.
Assessment
Pre-Activity Assessment
Guiding Questions: Get a sense of students’ pre-activity base knowledge by asking the class a few questions, such as the following:
- What are some famous arches? Have you seen arches used in your community?
- What advantages are provided when building with arches?
Activity Embedded Assessment
Calculations: As students build their model aqueducts, expect them to keep track of their calculations. Walk around and check that each group is doing the math and not guessing the dimensions. Have students hand in their calculations along with their completed model aqueducts.
Post-Activity Assessment
Project Evaluation: Evaluate students’ completed model aqueducts on the amount of effort and calculations put into the designs as well as whether the setups were successful in delivering water. Suggested grading guidelines include:
- Are the measurements accurate and documented?
- Did they incorporate the specified number of arches?
- Do the arches look uniform, neat and pleasing?
- Was the design successful at transferring the water from point A to B, with no loss of water?
- Did the water move at an adequate flow rate, not too fast (spilling out of the channel) or too slow (stagnant)?
Safety Issues
While testing the completed model aqueducts, watch that spills and excessive flow rate are avoided.
Troubleshooting Tips
If students have trouble building the arches due to the square building blocks, suggest that they put spacers (such as small folded pieces of paper) between each block before gluing them together.
Additional Multimedia Support
Show students two excellent artist’s renderings by O. Scarpelli of what a Roman aqueduct construction site might have looked like, including human workers, scaffolding, pulley systems to move materials and temporary formwork for building the arches at https://s-media-cache-ak0.pinimg.com/originals/16/79/88/167988c00e7ecf70a765d5c227c856f7.jpg and another at AncientVine.com, specifically http://ancientvine.com/lastmile.html.
Subscribe
Get the inside scoop on all things TeachEngineering such as new site features, curriculum updates, video releases, and more by signing up for our newsletter!More Curriculum Like This

Students see that geometric shapes can be found in all sorts of structures as they explore the history of the Roman Empire with a focus on how engineers 2000 years ago laid the groundwork for many structures seen today. Completing this lesson prepares students for the associated activity in which te...

Students are presented with a brief history of bridges as they learn about the three main bridge types: beam, arch and suspension. They are introduced to two natural forces — tension and compression — common to all bridges and structures.
Copyright
© 2016 by Regents of the University of ColoradoContributors
Nathan Coyle; Malinda Zarske; Andi Vicksman; Maia Vadeen; Ryan Sullivan; Russell Anderson; Lauchlin BlueSupporting Program
CU Teach Engineering (a STEM licensure pathway), Engineering Plus Degree Program, University of Colorado BoulderAcknowledgements
This activity was developed by CU Teach Engineering, a pathway to STEM licensure through the Engineering Plus degree program in the College of Engineering and Applied Science at the University of Colorado Boulder.
Last modified: July 21, 2023







User Comments & Tips