Quick Look
Grade Level: 12 (11-12)
Time Required: 45 minutes
Lesson Dependency:
Subject Areas: Physics
NGSS Performance Expectations:

| HS-PS3-2 |

Summary
Students begin to focus on the torque associated with a current carrying loop in a magnetic field. They solve example problems as a class and use diagrams to visualize the vector product. In addition, students learn to calculate the energy of this loop in the magnetic field. Through the associated activity, "Get Your Motor Running," students explore a physical model to gain empirical data and compare it to their calculated data. A homework assignment is also provided as a means of student assessment.Engineering Connection
Biomedical engineers must consider not only the force resulting from a charged particle in a magnetic field but also, a current traveling through a magnetic field. During resonance and relaxation, engineers can determine the frequency of the oscillating magnetic field based on a small current in the receiver coil of the MRI machine. In the homework assessment section of this lesson, students will have to determine the magnitude and direction of the torque on a current loop due to the magnetic field.
Learning Objectives
After this lesson students should be able to:
- Describe the actions of a current loop in a magnetic field.
- Draw and fully label a diagram of current acting through a loop.
- Calculate the torque on a current loop due to a magnetic field.
Educational Standards
Each TeachEngineering lesson or activity is correlated to one or more K-12 science,
technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN),
a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics;
within type by subtype, then by grade, etc.
Each TeachEngineering lesson or activity is correlated to one or more K-12 science, technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN), a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics; within type by subtype, then by grade, etc.
NGSS: Next Generation Science Standards - Science
| NGSS Performance Expectation | ||
|---|---|---|
|
HS-PS3-2. Develop and use models to illustrate that energy at the macroscopic scale can be accounted for as either motions of particles or energy stored in fields. (Grades 9 - 12) Do you agree with this alignment? |
||
| Click to view other curriculum aligned to this Performance Expectation | ||
| This lesson focuses on the following Three Dimensional Learning aspects of NGSS: | ||
| Science & Engineering Practices | Disciplinary Core Ideas | Crosscutting Concepts |
| Develop and use a model based on evidence to illustrate the relationships between systems or between components of a system. Alignment agreement: | Energy is a quantitative property of a system that depends on the motion and interactions of matter and radiation within that system. That there is a single quantity called energy is due to the fact that a system's total energy is conserved, even as, within the system, energy is continually transferred from one object to another and between its various possible forms. Alignment agreement: At the macroscopic scale, energy manifests itself in multiple ways, such as in motion, sound, light, and thermal energy.Alignment agreement: These relationships are better understood at the microscopic scale, at which all of the different manifestations of energy can be modeled as a combination of energy associated with the motion of particles and energy associated with the configuration (relative position of the particles). In some cases the relative position energy can be thought of as stored in fields (which mediate interactions between particles). This last concept includes radiation, a phenomenon in which energy stored in fields moves across space.Alignment agreement: | Energy cannot be created or destroyed—it only moves between one place and another place, between objects and/or fields, or between systems. Alignment agreement: |
International Technology and Engineering Educators Association - Technology
-
Energy can be grouped into major forms: thermal, radiant, electrical, mechanical, chemical, nuclear, and others.
(Grades
9 -
12)
More Details
Do you agree with this alignment?
-
Assess how similarities and differences among scientific, mathematical, engineering, and technological knowledge and skills contributed to the design of a product or system.
(Grades
9 -
12)
More Details
Do you agree with this alignment?
State Standards
National Science Education Standards - Science
-
Results of scientific inquiry--new knowledge and methods--emerge from different types of investigations and public communication among scientists. In communicating and defending the results of scientific inquiry, arguments must be logical and demonstrate connections between natural phenomena, investigations, and the historical body of scientific knowledge. In addition, the methods and procedures that scientists used to obtain evidence must be clearly reported to enhance opportunities for further investigation.
(Grades
9 -
12)
More Details
Do you agree with this alignment?
Worksheets and Attachments
Visit [www.teachengineering.org/lessons/view/van_mri_lesson_3] to print or download.Pre-Req Knowledge
Students need to have an understanding of rotation and torque, since an important concept of this lesson is the torque created by a magnetic field. Students need to make the connection that torque on the loop leads the loop to rotate in the magnetic field. It is also crucial for the student to draw and label all example and homework problems (just as shown in the figure sets).
Introduction/Motivation
We are making progress to solve the MRI challenge problem and determine the risks and dangers of an MRI. To do this, we need to learn what happens near a large electromagnet. We need to learn about the properties of magnetic fields, including the effects of a magnetic field on loops of current. As MRI machines evolved over time and were built with stronger magnets, engineers needed to account for the change in current. Refer to the associated activity Get Your Motor Running for students to investigate real applications such as electro motors used in modern products.
Lesson Background and Concepts for Teachers
Legacy Cycle Information
This lesson fits into the Research and Revise phase of the legacy cycle in which students are provided with additional information enabling them to revise their initial ideas for solving the challenge. The research aspect consists of formal lecture and several example problems on how magnetic fields create torque on a current loop.
Torque on a Current Loop
Note: It may be helpful to review rotation, torque and kinetic energy at this point.
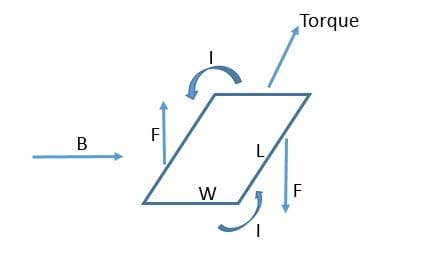
Consider a rectangular loop of current in a magnetic field with width w and length l, and current I. Let the loop be laid out on a horizontal plane and let a magnetic field B point directly to the right. (see Figure A)
First, the field will exert no force on the two width segments of the wire as in this length the current is parallel to the magnetic field. However, there will be a force on each of the lengths of the rectangular loop as the current is perpendicular to the field on that segment. The magnitude of these forces is:
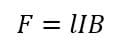
Notice that these two forces are equal and opposite in magnitude, so there is no translational movement, but there is a torque about the center of the loop. The two torques from the forces can be calculated as:
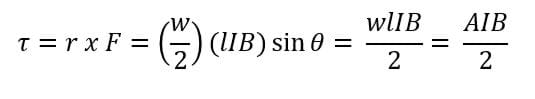
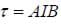
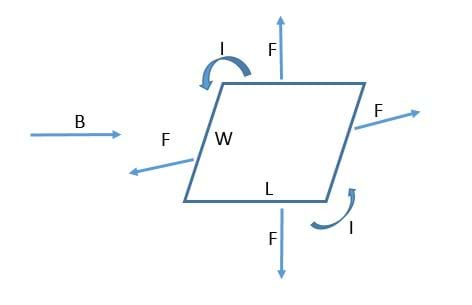
Once the loop is fully rotated, the forces appear in the configuration shown to the right. Notice that there is no longer any torque, but outward force in every direction. The forces on the width elements never contribute to the torque, but rather attempt to stretch the loop. At any angle during the rotation, the torque could be written as:

Generalizing further, imagine if the current loop actually consisted of N turns. While this would have no effect on the purely electrical properties of the circuit (assuming no resistance in the wire) the amount of current contributing to the magnetic force on the wire would be effectively multiplied by N. Thus,
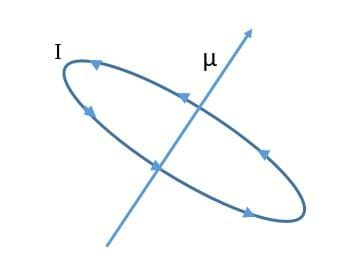
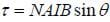
We will define the magnetic moment of the current loop as a vector normal to the plane of the loop directed according to the right hand rule with respect to the current (see diagram). The magnitude of this vector will be NAI , and the vector will be denoted μ. With this definition, we can write:
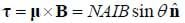
This can be shown to work if the loop is not rectangular, and is valid for any loop in a plane. Note that the torque will accelerate the loop so that the magnetic moment will become parallel to the field.
Example:
A circular wire loop of 5 turns with a radius of 10 cm weighing 54 g with a current of 1.3 A is lying flat on a table. A strong uniform magnetic field of 3 T is projected up 30 degrees from the horizontal, as shown in the diagram. Find the magnitude of the torque on the current loop.
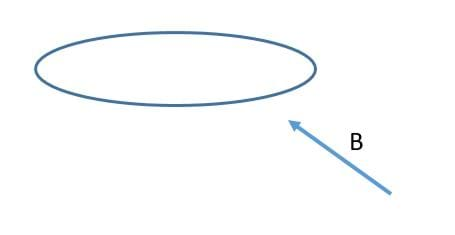
Solution: Start by drawing the picture and labeling accordingly!
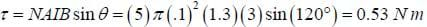
Note that the gravitational torque on this loop is 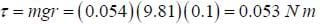
So the magnetic torque will rotate part of the loop off the table!
Energy of a Current Loop in a Magnetic Field
When a current loop is rotated through an (infinitesimally) small angle 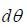
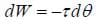
Then the decrease in potential energy is
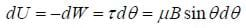
And so by integrating
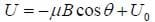
Since it is only the change in potential energy that we are ever interested in, we can simply make a convention for when the potential energy is zero, and get rid of 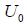
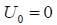
Then,

Note that the potential energy is lowest when μ and B are parallel and there is no torque. It is highest when μ and B are antiparallel. In the antiparallel case, a slight nudge in either direction will create a torque sending it back towards the parallel position.
Example:
A solid hoop of copper with radius 35 cm weighing 200 g is bolted to a stand so that it may rotate freely as shown in the diagram below. A current of 5.5 A is sent around the hoop, and a uniform magnetic field of 2000 Gauss is directed up. Describe the motion of the hoop. What is the maximum kinetic energy of the hoop? What is the maximum angular velocity?
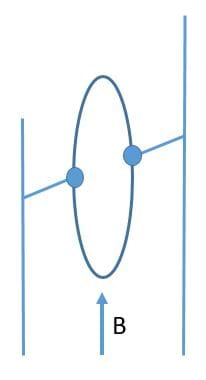
Solution: Start by drawing the picture and labeling accordingly!
The hoop will oscillate back and forth, with a maximum kinetic energy when the hoop is oriented horizontally and will momentarily halt whenever it is vertical.
The initial potential energy is zero, and the minimum potential energy when the magnetic moment lines up with the field is
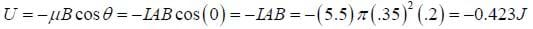
So the maximum kinetic energy is 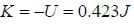
The moment of inertia of the hoop is 
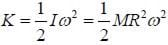
Thus

This is very close to 1 full rotation per second!
As a final note, one may notice that permanent magnets such as a compass needle have a tendency to rotate into the plane of a field, suggesting that they are comprised of a current loop. In fact, a permanent magnet can be explained as a collection of many tiny current loops, and from this model we will be able to explain the phenomena of attraction and repulsion.
Associated Activities
- Get Your Motor Running - Students investigate motors and electromagnets as they construct their own simple electric motors using batteries, magnets, paper clips and wire.
Assessment
Homework: Assign students to complete the Current Loops in Magnetic Fieilds Homework set, which serves as an assessment tool.
Subscribe
Get the inside scoop on all things TeachEngineering such as new site features, curriculum updates, video releases, and more by signing up for our newsletter!More Curriculum Like This

Students induce EMF in a coil of wire using magnetic fields. Students review the cross product with respect to magnetic force and introduce magnetic flux, Faraday's law of Induction, Lenz's law, eddy currents, motional EMF and Induced EMF.

After a demonstration of the deflection of an electron beam, students review their knowledge of the cross-product and the right-hand rule with example problems. Students apply these concepts to understand the magnetic force on a current carrying wire. Through the associated activity, students furthe...

Beginning with a class demo, students are prompted to consider how current generates a magnetic field, and the direction of the field that is generated. Via a lecture, students learn Biot-Savart's law (and work some sample problems) in order to calculate, most simply, the magnetic field produced in ...

A class demo introduces students to the force between two current carrying loops, comparing the attraction and repulsion between the loops to that between two magnets. After a lecture on Ampere's law (including some sample cases and problems), students begin to use the concepts to calculate the magn...
Copyright
© 2013 by Regents of the University of Colorado; original © 2006 Vanderbilt UniversityContributors
Eric AppeltSupporting Program
VU Bioengineering RET Program, School of Engineering, Vanderbilt UniversityAcknowledgements
The contents of this digital library curriculum were developed under National Science Foundation RET grant nos. 0338092 and 0742871. However, these contents do not necessarily represent the policies of the NSF, and you should not assume endorsement by the federal government.
Last modified: September 20, 2020







User Comments & Tips