Quick Look
Grade Level: 8 (7-9)
Time Required: 45 minutes
Lesson Dependency:
Subject Areas: Algebra
Summary
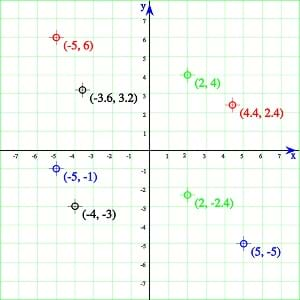
A brief refresher on the Cartesian plane includes how points are written in (x, y) format and oriented to the axes, and which directions are positive and negative. Then students learn about what it means for a relation to be a function and how to determine domain and range of a set of data points from the modeling game found in the associated activity.Engineering Connection
Many important engineering relationships are easily understood in the form of graphs. Graphing is essential to understanding the mathematics involved in all types of engineering. For example, civil engineers must understand graphing to be able to determine certain areas of stresses and strain within the building plans for bridges and other structures. In journal questions 1-5 (in the Assessment section), students consider the importance of creating visual representations of data, as well as possible data sources.
Learning Objectives
After this lesson, students should be able to:
- Describe the Cartesian plane and correctly label its parts.
- Explain the source of the name "Cartesian."
- Describe the naming convention for coordinates in the form (x, y).
- Explain what a function is and how to tell if a set of coordinates is a function.
- Determine the domain and range of a set of points.
- Plot a set of data points.
- Explain how understanding graphing will help with solving the challenge.
Educational Standards
Each TeachEngineering lesson or activity is correlated to one or more K-12 science,
technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN),
a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics;
within type by subtype, then by grade, etc.
Each TeachEngineering lesson or activity is correlated to one or more K-12 science, technology, engineering or math (STEM) educational standards.
All 100,000+ K-12 STEM standards covered in TeachEngineering are collected, maintained and packaged by the Achievement Standards Network (ASN), a project of D2L (www.achievementstandards.org).
In the ASN, standards are hierarchically structured: first by source; e.g., by state; within source by type; e.g., science or mathematics; within type by subtype, then by grade, etc.
Common Core State Standards - Math
-
Use a pair of perpendicular number lines, called axes, to define a coordinate system, with the intersection of the lines (the origin) arranged to coincide with the 0 on each line and a given point in the plane located by using an ordered pair of numbers, called its coordinates. Understand that the first number indicates how far to travel from the origin in the direction of one axis, and the second number indicates how far to travel in the direction of the second axis, with the convention that the names of the two axes and the coordinates correspond (e.g., x-axis and x-coordinate, y-axis and y-coordinate).
(Grade
5)
More Details
Do you agree with this alignment?
-
Understand signs of numbers in ordered pairs as indicating locations in quadrants of the coordinate plane; recognize that when two ordered pairs differ only by signs, the locations of the points are related by reflections across one or both axes.
(Grade
6)
More Details
Do you agree with this alignment?
-
Find and position integers and other rational numbers on a horizontal or vertical number line diagram; find and position pairs of integers and other rational numbers on a coordinate plane.
(Grade
6)
More Details
Do you agree with this alignment?
-
Understand that a function is a rule that assigns to each input exactly one output. The graph of a function is the set of ordered pairs consisting of an input and the corresponding output.
(Grade
8)
More Details
Do you agree with this alignment?
-
Understand that a function from one set (called the domain) to another set (called the range) assigns to each element of the domain exactly one element of the range. If f is a function and x is an element of its domain, then f(x) denotes the output of f corresponding to the input x. The graph of f is the graph of the equation y = f(x).
(Grades
9 -
12)
More Details
Do you agree with this alignment?
-
Use function notation, evaluate functions for inputs in their domains, and interpret statements that use function notation in terms of a context.
(Grades
9 -
12)
More Details
Do you agree with this alignment?
-
Relate the domain of a function to its graph and, where applicable, to the quantitative relationship it describes.
(Grades
9 -
12)
More Details
Do you agree with this alignment?
International Technology and Engineering Educators Association - Technology
-
Explain how knowledge gained from other content areas affects the development of technological products and systems.
(Grades
6 -
8)
More Details
Do you agree with this alignment?
-
Use devices to control technological systems.
(Grades
6 -
8)
More Details
Do you agree with this alignment?
Worksheets and Attachments
Visit [www.teachengineering.org/lessons/view/van_linear_eqn_less2] to print or download.Pre-Req Knowledge
Familiarity with the coordinate plane, coordinates and equations are helpful but not required.
Introduction/Motivation
(In advance, make copies of the Work It Out Worksheet.Then prepare to shows students the 11-slide Linear Functions Presentation PowerPoint® file, which provides notes for them. The presentation is composed of three sections, delineated by three different slide background colors [blue, gray, gold]. The slides are "animated," so click the mouse [or space bar] to make the next items appear. Show slides 1-4 with the Introduction/Motivation section. Show slides 4-8 when presenting the Lesson Background information. Use slides 9-11 in conjunction with the associated activity, Club Function; they are included in this lesson presentation as a teacher preview of the activity.)
(While showing slides 1-4, present the following information to students.)
Have you ever liked two things so much that you wanted to combine them to make one amazing thing? (Listen to student comments.) For example, think about peanuts and chocolate chips. Combined together, they can make peanut butter cookies with chocolate chips on top! Or what about the game of Slamball? It combines the fun of jumping on a trampoline with the rules of basketball. Both great ideas, and there are many more.
One interesting combination has to do with a French man who really liked math back in the 1600s. His name was Rene Descartes, and he liked both algebra and geometry, but back then people did not think those two topics were very much related. Decartes started looking for ways to combine them so that they could be used together for important applications. He came up with this neat way of taking numbers that belong in the realm of algebra and plotting them visually onto a geometric coordinate plane to show how they are related. This coordinate plane became known as the "Cartesian plane," named after him.
Several parts of the coordinate plane are important to understand before we can learn how to use it. In today's lesson, you will learn about it and how it is used.
(Hand out the worksheets and continue on to present students with the Lesson Background content.)
Lesson Background and Concepts for Teachers
While still showing slide 4 of the attached PPT file, cover the following information with students, as they make notes on the worksheet:
- Take students through labeling the axes with "x" and "y," as well as adding arrows to the ends of each axis to show it goes on forever.
- Talk about the Cartesian plane quadrants, having students label them "I, II, III and IV."
- Point out which directions are positive (right on the x-axis and up on the y-axis) and which directions are negative (left on the x-axis and down on the y-axis), and have students mark out scales on each axis.
- Point out the origin, the point (0,0).

While still showing slide 4, begin talking about coordinates: What does (0,0) mean? Students may say moving to 0 units to the left or right on the x-axis and moving 0 units up or down on the y-axis. Prompt them further to tell you which is x and which is y. Then tell them that we can generally write the format as (x,y), and have them fill that in on their worksheets.
Go through the process of plotting a couple of points with them. Depending on their level of familiarity with the topic, little prompting may be necessary. When they understand the concept, have them practice plotting the coordinates on the bottom of their worksheets. Then review the answers as a class and clarify any misconceptions. (This is a good stopping place for shorter class periods, as students can finish plotting points as homework.)
While showing slides 5 – 8, teach students the definition of a function. Use the slides to define relations, functions, domain, range, and linear functions. Have students do the practice problems on slide 8 and discuss their answers as a class.
For homework, assign students to complete the worksheet (if not done in class) and the Lesson 2 Practice Sheet Homework on the coordinate plane, function, domain and range.
Note: Slides 9 – 11 explain the Club Function game activity rules (only included here for teacher preview). Refer to the associated Club Function activity for detail and examples.
Associated Activities
- Club Function - Students learn about relations and functions through an interactive modeling game.
Assessment
Practice: During the lesson (or as homework), have students complete the Work It Out Worksheet to practice plotting some coordinates on their own.
Journal Questions: At lesson end, have students create in their journals small graphs of the data points from the grand challenge data set. Graph the first 10 points. Create the appropriate scale and approximate the location of each point. Then have them write answers to the following questions.
- Do you see a trend forming?
- Draw a line approximating this trend.
- What did this graph tell you that you did not already know from looking at the data?
- Why is graphing a set of data important?
- Where do you think this data could have come from?
Homework: Assign students to complete the Lesson 2 Practice Sheet Homework on coordinate plane, functions, domain and range to give them practice determining whether a group of points is a function, and determining the domain and range of sets of points.
Subscribe
Get the inside scoop on all things TeachEngineering such as new site features, curriculum updates, video releases, and more by signing up for our newsletter!More Curriculum Like This

Students explore the definition of a function by playing an interactive game called "Club Function." Through this activity students come to understand that one x-coordinate can only have one corresponding y-coordinate while y-coordinates can have many x-coordinates that correspond to it.

Students learn about the first attempts at machine learning and specifically about the perceptron model—a simplified model of a biological neuron.

Students learn about linear programming (also called linear optimization) to solve engineering design problems. They apply this information to solve two practice engineering design problems related to optimizing materials and cost by graphing inequalities, determining coordinates and equations from ...

Students learn about an important characteristic of lines: their slopes. Students get an explanation of when and how these different types of slope occur.
Copyright
© 2013 by Regents of the University of Colorado; original © 2007 Vanderbilt UniversityContributors
Aubrey McKelveySupporting Program
VU Bioengineering RET Program, School of Engineering, Vanderbilt UniversityAcknowledgements
The contents of this digital library curriculum were developed under National Science Foundation RET grant nos. 0338092 and 0742871. However, these contents do not necessarily represent the policies of the NSF, and you should not assume endorsement by the federal government.
Last modified: May 23, 2019








User Comments & Tips